<< Back to BIEN 435 Course Rules
Young’s Modulus of a
Chicken Bone
Introduction
The
strength of bone depends on the stresses to which it is subjected. While we have not done a biomechanical
analysis of a leg and thigh bone of a chicken, we can suggest that the two may
be subjected to different stresses and may therefore have different
properties. This laboratory is designed
to examine the elastic modulus of the chicken leg and thigh bone.
Null Hypothesis
The null
hypothesis for this laboratory is:
The Young's modulus of a chicken leg bone (scientific term “ossius drumstickus”) is the same
as that for a chicken thigh bone.
The
alternative hypothesis is:
The Young’s modulus of a chicken leg bone differs from that
of a chicken thigh bone.
Experimental Setup
Again, you
will assemble your own experimental system.
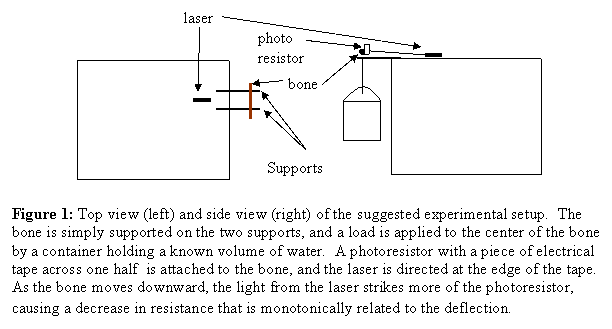
You can
support the bone so that it looks like a simply supported beam, as depicted in
Figure 2.
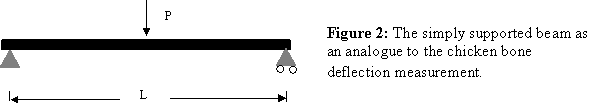
is
the load applied to the middle of the beam (bone), and 
is the distance
between the supports (not the length of the bone). The load pushes the beam downward by a small amount 
, according to the following formula:
The
equation can be solved for 
as a function of and .
Thus, by
measuring both load and deflection you can back E out of the equation.
Transducer Calibration
You will
need to calibrate your displacement detector, based on some known displacement. The concept for the displacement transducer
is shown in Figure 3. A photoresistor with a piece of electrical tape across one half is attached to
the bone, and the laser is directed at the edge of the tape. As the bone moves downward, the light from
the laser strikes more of the photoresistor, causing
a decrease in resistance that is monotonically related to the deflection.
Applied Load
You need to
ensure that you know how much load was applied to your bone. Calibrated weights would serve this purpose
well. An alternative is to use water as
a standard. You know the density of
water (1 g/cm3 in the metric scale, or, in the English system “a
pint’s a pound the world around”). Thus,
if you can measure a volume of water, you know the mass. Of course, density
will vary somewhat with temperature, but is the variation significant for the
purpose of this laboratory?
You will
want to measure deflection for a number (N) of applied loads.
The I Parameter
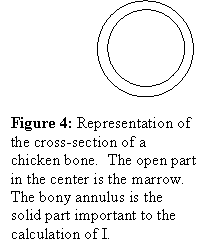The equation for deflection as a
function of load includes the parameter I, which depends on the geometry of the
beam (bone). You should make the
assumption that the bone is a hollow tube, with a cross-section as shown to the
right. You will need to know the
thickness of the wall and the bone outer or inner diameter.
Data analysis
1. Determine the calibration of your
sensor (concentration as a function of voltage).
2. Determine the applied loads for each
of the N measurements.
3. From your sensor and its
calibration, determine the deflection for each applied load.
4. Plot deflection as a function of
applied load.
5. Use the equation from your statics book to determine the elastic modulus, given the
data you collected for each of the N pairs of deflection and load.
6. When you have obtained the elastic
modulus (the average of the N trials), you can use this to compare theory (the
equation for deflection as a function of load) to the data you collected.
7. Discuss reasons for any differences
(theory vs experiment).
1. Perform Student’s T-tests to test
the hypothesis. Provide a p-value. Are the results significant?
BIEN 435
Last Updated February 27, 2005
Steven A. Jones