Physiological Modeling

Recall the cardiac output problem from last quarter:

To measure cardiac output, a physician inserts a catheter into the left atrium, injects saline at room temperature, and measures the temperature in the aorta. The situation can be modeled as below:

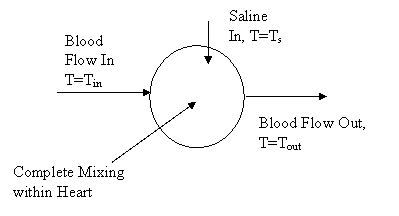
You wish to know:

1. The temperature T_out as a function of time, assuming that the saline inflow is constant for a given period of time (t_in) and zero otherwise.
2. The relationship between the area under the curve (T vs. time) and the flow rate.

Consider assumptions that are made in clinical applications:

1. Saline is injected continuously at a constant rate over a few seconds (t_s).
2. Inflow from the vena cava is constant in time.
3. Outflow to the aorta is constant in time.
4. All heat loss from the heart is a result of inflow and outflow. I.e., the circulatory system is adiabatic.
5. There is complete mixing within the heart.
6. Inflow and outflow profiles are “plug flow.”
7. Temperature of the blood from the vena cava is constant.

None of these assumptions is true. For each assumption, state why it is not true. Then provide a reasonable argument as to why it can be considered “approximately true.”