LAB 7- Convection Heat Transfer from a Flat Plate

Introduction

One of the fundamental problems of interest in convection heat transfer considers flow over a heated flat plate. While the geometry could not be any more basic, parallel flow over a flat plate, as shown in Figure 1, occurs in a number of engineering applications. In addition, this geometry may be a good first approximation for flow over surfaces that are slightly contoured, such as airfoils or turbine blades. In this type of external flow, the boundary layers develop freely, without any constraints imposed by adjacent surfaces. Consequently, there will always be a region of the flow outside the boundary layer in which velocity and temperature gradients are negligible.

Figure 1. Parallel Flow Over a Flat Plate

By nondimensionalizing the boundary layer conservation equations, it can be shown that the local and average convection coefficients may be correlated by equations of the form

Nu_x = f₁(x*, Re_x, Pr)        (1)

  (2)

where Nux = Nusselt number (h_x/k_f)
Re_x = Reynolds number (ux/)
Pr = Prandtl number
x* = dimensionless axial location (x/L)
h = heat transfer coefficient
k_f = thermal conductivity of the fluid
u = free stream velocity
= kinematic viscosity

Note that the properties of the fluid are based on the film temperature and that the subscript x has been appended to the Nusselt number to emphasize that the interest here is in local conditions on the surface. The overbar indicates an average value of the Nusselt number from x* = 0 to the point of interest. One of the primary objectives of convection heat transfer is to find the functions f₁ and f₂. Either theoretical or experimental approaches could be used for this purpose.

Two boundary conditions that are frequently used in heat transfer analysis are 1) uniform surface temperature and 2) uniform surface heat flux. For a uniform heat flux boundary condition on a flat plate exposed to forced convection flow, integral methods [1] may be used to determine the local Nusselt number for laminar flow. The correlation that results is

(3)

In a similar fashion, it can be shown that for turbulent flow

(4)

When compared to the results for a uniform surface temperature, these solutions give values of Nux that are 36 % and 4% larger for laminar and turbulent flow, respectively.

It is quite common to have a situation where the leading edge of the flat plate is unheated, otherwise known as an unheated starting length. In this case, the surface temperature of the unheated section equals that of the fluid (Ts = T_f). As shown in Figure 2, the velocity boundary layer begins to develop at the leading edge (x = 0), while the thermal boundary layer development starts at x = . An integral boundary layer solution can be used [1] to develop an expression for the local Nusselt number that accounts for the unheated length. The equation for laminar flow is

      (5)

where  is given by Equation 3. Similarly, the unheated starting length affect on heat transfer from a flat plate experiencing turbulent flow can be expressed as

      (6)

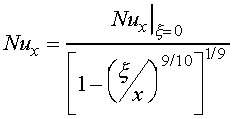
where  is given by Equation 4. The equations for the unheated starting length apply for x > only. According to Incropera and DeWitt (1990), in order to obtain average Nusselt numbers for  < x < L, these equations would have to be integrated numerically.

Figure 2. Flat Plate in Parallel Flow with an Unheated Starting Length

Objectives

The primary objective of this exercise is to measure the local heat transfer coefficient and Nusselt number for a heated flat plate experiencing forced convection cooling. The plate has a short unheated starting length. The measured data is to be compared to theoretical predictions.

Experimental Apparatus

The flat plate for this experiment consists of a smooth rectangular surface, 18 inches long by 11 1/2 inches wide with a metal rectangular plate (10 inches by 5-1/2 inches) embedded in it. The metallic plate is located such that there is a 6 inch unheated starting length.  Surface temperatures of the metallic plate may be monitored by 15 type-T thermocouples installed along the center of the plate in the flow direction. These thermocouples are located at 0.625 inch increments starting at 0.625 inches from the front edge of the metallic plate.  The thermocouples are connected to a data acquisition system that provides the temperatures of the thermocouples on a computer screen. A film heater is embedded in the metallic plate and is connected to a DC power supply. The total power to the heater may be determined from measurements of the total voltage supplied to the heater and the total current to the heater determined from the voltage drop across a 10 mW shunt. Assume the power supplied to the heater is divided equally between the top and bottom surface of the metallic plate. A dimensioned drawing of the flat plate apparatus is shown in Figure 3.

Figure 3. Heated Flat Plate

The AEROLAB Educational Tunnel supplies the forced air for the experiment. The flat plate apparatus has been installed such that the flat surface is centered within the 12 inch square test section and air flows over the top surface as well as the bottom surface of the flat plate. A variable speed motor drives the wind tunnel centrifugal fan, allowing different free stream velocities to be tested. The free stream air speed within the wind tunnel is displayed on the wind tunnel control panel in mph.

Newton's Law of Cooling may be used to calculate the local heat transfer coefficient and Nusselt number. This equation may be written as

      (7)

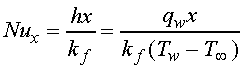
where q_w is the heat flux per unit area.

Experimental Procedure

1. Determine the local barometric pressure and temperature.
2. Turn on the power to the film heater. The lab instructor will specify the setting for the power supply.
3. Turn on the power to the wind tunnel and adjust the motor speed control in order to obtain the desired flow rate. The lab instructor will once again suggest an appropriate flow rate. Allow the flow to attain a steady state condition.
4. Monitor and record the free steam temperature and velocity and the plate temperatures over time. Continue until a steady state condition is achieved. Data acquisition is accomplished with a Keithley Series 500 Data Acquisition and Control system. Most of the important data can be read from the computer monitor and may also be printed when desired.
5. At steady state, record the following: all plate temperatures, total voltage to the heater, voltage drop across the shunt (or the current supplied to the heaters), air speed, and air temperature.

Calculate the wind tunnel Reynolds number. Determine the heat supplied to each plate. Find local values of h_x, Re_x, and Nu_x. Compare the experimentally determined parameters with the theoretical predictions. A graphical representation of the results is desirable.

1. Consider the heat loss from the plate due to radiation to the surroundings and by conduction through the edges of the flat plate apparatus. Make estimates of these losses and discuss means of minimizing or accounting for them. Do these losses help to explain any discrepancies that are observed between the experimental and theoretical data?
2. Is the uniform heat flux boundary condition a good approximation for this experiment?
3. How does the experimental data agree with the external flow assumption that the hydrodynamic and thermal boundary layers develop freely? Does the presence of the wind tunnel walls affect the boundary layer development along the flat plate?
4. Discuss how the heat transfer coefficient varies for flow over a flat plate through the laminar, transition , and turbulent flow regimes. Based on your results, did transition from a laminar to a turbulent boundary layer occur for your test? If so, determine the distance from the leading edge where the experimental flow became turbulent. Compare to the theoretical value and discuss any discrepancies.
5. Determine the uncertainty in the calculated values of Nu_x for your experiment.

1. Kays, W.M., and Crawford, M.E., Convective Heat and Mass Transfer, McGraw-Hill, New York, 1980.
2. Incropera, F.P. and DeWitt, D.P., Fundamentals of Heat and Mass Transfer, 3rd ed., John Wiley & Sons, New York, 1990.
3. Holman, J.P., Heat Transfer, 7th Ed., McGraw-Hill Book Co., New York, 1990.
4. White, F.M., Fluid Mechanics, 2nd Ed., McGraw-Hill Book Co., New York, 1986.
5. Chappa, S.C. and Canale, R.P., Numerical Methods for Engineers, 2nd Ed., McGraw-Hill, New York, 1988.