ME
354 - Thermofluids Laboratory
Spring 1997
LAB 8 - Pool Boiling Heat Transfer
Introduction
Boiling is the term used to describe the phenomena that occurs when
evaporation takes place at a solid-liquid interface. The process will occur
when the surface temperature Ts exceeds the saturation
temperature associated with the liquid pressure Tsat.
Heat is transferred to the liquid from the surface according to Newton's
law of cooling
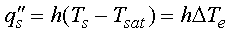 (1)
(1)
where 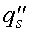 = heat flux at the surface
= heat flux at the surface
h = heat transfer coefficient
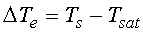 , excess temperature
, excess temperature
Boiling is characterized by the formation of vapor bubbles, which grow
and eventually disengage from the surface. The growth of the bubbles and
their subsequent dynamics depend upon the excess temperature, the surface
characteristics, and on the thermophysical properties of the fluid, the
most important of which is the surface tension. The dynamics of the vapor
bubble formation influence fluid motion near the surface which affects
the heat transfer coefficient.
Boiling may transpire under a variety of conditions. In this experiment,
pool boiling will be investigated. This term refers to a condition
where the liquid is quiescent and the liquid motion near the surface is
due to free convection and to mixing induced by bubble growth and subsequent
detachment. Another definition for pool boiling refers simply to
conditions in which the heated surface is submerged below a free surface
of liquid. Saturated boiling is a condition in which most of the
liquid is at a temperature slightly greater than saturation while at the
liquid-solid interface there is a significant decrease in liquid temperature.
Bubbles that form on the solid surface are driven upward by buoyant forces
and eventually are transported to the gas above the free surface. Figure
1 depicts the typical temperature distribution in saturated pool boiling.
Subcooled boiling occurs when the liquid temperature is less than
the saturation temperature and the bubbles that are formed eventually condense
in the liquid.
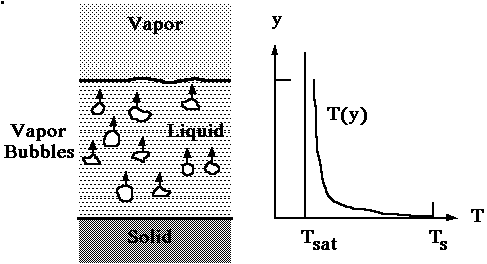 Figure 1. Saturated pool boiling temperature distribution
Figure 1. Saturated pool boiling temperature distribution
Saturated pool boiling has received a considerable amount of attention
within the heat transfer community. In this experiment the primary interest
is the boiling curve, which considers the effect of excess temperature
on either the heat transfer coefficient or the heat flux. By studying the
different modes, or regimes, of saturated pool boiling, an appreciation
for the underlying physical mechanisms may be obtained. These regimes are
identified in the boiling curve of Figure 2. The curve shown is for water,
but similar trends are observable in other fluids. Equation 1 indicates
that 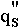 depends
upon both the heat transfer coefficient h and the excess temperature. The
various boiling modes can be differentiated according to the value of excess
temperature. The different regimes evident on the boiling curve include
film boiling, transition boiling, nucleate boiling, and free convection
boiling. A more complete description of pool boiling and the boiling regimes
can be found in Holman (1990).
depends
upon both the heat transfer coefficient h and the excess temperature. The
various boiling modes can be differentiated according to the value of excess
temperature. The different regimes evident on the boiling curve include
film boiling, transition boiling, nucleate boiling, and free convection
boiling. A more complete description of pool boiling and the boiling regimes
can be found in Holman (1990).
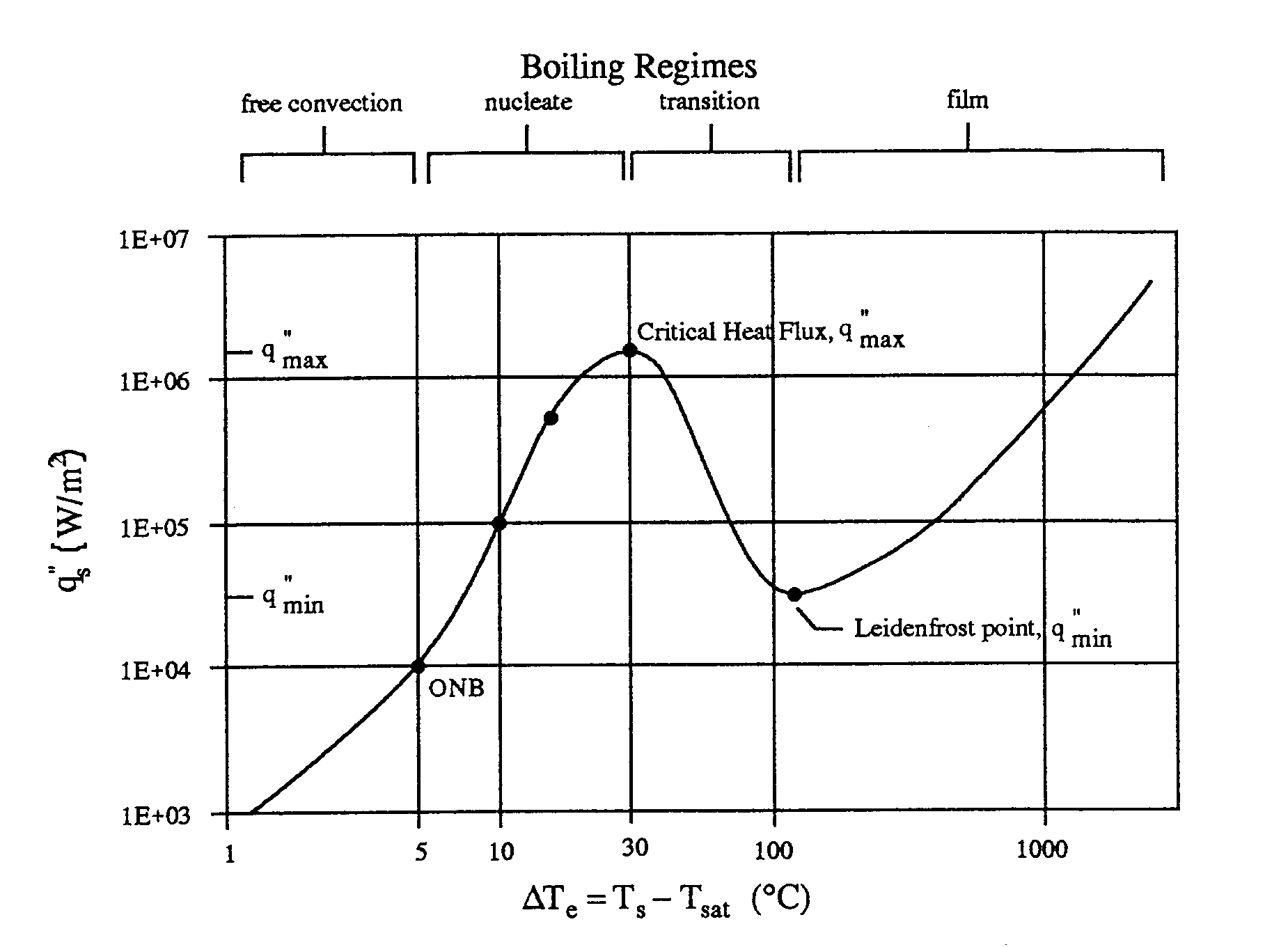 Figure 2. Typical pool boiling curve for water at 1 atmosphere
Figure 2. Typical pool boiling curve for water at 1 atmosphere
Objectives
Pool boiling will be investigated in this exercise by immersing an aluminum
cylinder, initially at room temperature, into a container of liquid nitrogen.
The primary objective is to generate a pool boiling curve similar to that
in Holman (1990), where h is given as a function of the excess temperature 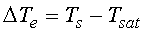 .
In addition, the different boiling regimes are to be observed, including
any interesting physical phenomena, and then reported and discussed in
the laboratory report.
.
In addition, the different boiling regimes are to be observed, including
any interesting physical phenomena, and then reported and discussed in
the laboratory report.
Experimental Apparatus
A sufficient supply of liquid nitrogen, such that the aluminum cylinder
may be completely immersed, will be contained in a glass dewar flask. Most
dewar flask are made from metals; however, the glass flask will allow the
boiling process to be observed. Each student group will be provided with
a test cylinder which is approximately 1.5" in diameter and 1.5" in length.
A single type-T thermocouple is imbedded in the aluminum cylinders. The
cylinders will be suspended from a lab stand at a height that allows the
cylinders to be fully immersed in the liquid N2.The
thermocouple emf will be read by a Keithley Series 500 data acquisition
system supplied with an Aim 6 thermocouple data acquisition card.
A computer program using SOFT500 has been written that will allow the data
acquisition system to read the thermocouple over the course of the experiment.
Time and temperature data will be taken at approximately 1 second intervals
for about 12 minutes. The data should be written to a file on the B: drive,
which, on the supplied 286 computer, requires a 3 1/2" low density diskette
(EACH GROUP MUST BRING A FORMATTED LOW DENSITY DISKETTE TO COLLECT THEIR
DATA).
The required thermophysical properties of aluminum are temperature dependent.
Data for thermal conductivity and specific heat of aluminum have been fit
to a 3rd order polynomial equation in temperature. The general equation
has the form
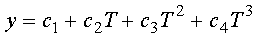 (2)
(2)
where T = temperature (°K). Table 1 lists the values of the constants
for the two properties of interest.
Table 1. Constants used in Equation 2
|
|
Thermal Conductivity
|
Specific Heat
|
|
|
W/m/°C
|
kJ/kg/°K
|
| c1
|
0.3893171E+03
|
-0.3973402E+00
|
| c2
|
-0.2870259E+01
|
0.1266628E-01
|
| c3
|
0.1367150E-01
|
-0.4477521E-04
|
| c4
|
-0.2054480E-04
|
0.5673365E-07
|
The lumped-heat-capacity method may be implemented in order to determine
the heat transfer coefficient as a function of excess temperature. The
underlying assumption in this analysis of transient heat conduction is
that the entire system is uniform in temperature or, in other words, that
the internal resistance is negligible in comparison with the external resistance.
Applying the first law of thermodynamics to the body being cooled results
in
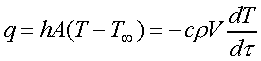 (3)
(3)
where 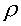 = density
= density
c = specific heat
V = volume
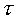 = time
= time
A = surface area for convection
T = temperature of lumped mass
Safety Precautions
Each student must have safety glasses in order to participate in the laboratory
exercise. Exercise caution around the liquid N2. Exposing
skin to the cold temperature liquid may cause burns.
Procedure
-
Determine the local barometric pressure. Weigh the aluminum cylinder
to used in the test. Determine the channel number the thermocouple is connected
to on the Keithley data acquisition card.
-
Start the data acquisition program and follow its instructions.
-
Lower the cylinder into the liquid N2 and begin
data acquisition.
-
Record time and temperature data at 1 second intervals until the excess
temperature nears zero and you are sure that the boiling regime is entirely
free convection. While boiling is occurring, closely observe and record
all phenomena of interest for the entire boiling curve. Particular attention
should be directed toward observing the transition from film boiling to
nucleate boiling that occurs very rapidly (in 2 or 3 seconds) and is accompanied
by very rapid, noisy boiling.
-
Remove the cylinder from the liquid N2. The program
will write all of the data to your disk..
Data Reduction and Points of Interest
-
Construct the boiling curve for aluminum in liquid N2.
Discuss the experimental boiling curve by comparing it to other empirical
results in the literature.
-
Discuss all of the phenomena that you observed for the various boiling
regimes. Relate to the boiling curve produced from the data.
-
Discuss the effect that the attached thermocouple has on the experiment.
Suggest means of reducing these effects.
-
Discuss the validity of the lumped-heat-capacity assumption for this
heat transfer process. Try to use quantitative arguments for this discussion.
References
-
Holman, J.P., Heat Transfer, 7th Ed., McGraw-Hill Book Co., New York,
1990.
-
Incropera, F.P. and Dewitt, D.P., Fundamentals of Heat and Mass Transfer,
John Wiley & Sons, New York, 1990.
-
Chappa, S.C. and Canale, R.P., Numerical Methods for Engineers, 2nd
Ed., McGraw-Hill, New York, 1988.
-
Yakowitz,S., and Szidarovszky, F., An Introduction to Numerical Computations,
2nd Ed., Macmillan Publishing Co., New York, 1989.
-
Holman, J.P., Experimental Methods for Engineers, 4th Ed., McGraw-Hill
Book Co., New York, 1984.



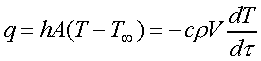 (3)
(3)